Born Effective Charges Yield Novel Interpretation of Drude Weight
Dr. Cyrus Dreyer and collaborators have found new results for Born effective charges (BECs) in conductors. BECs, defined as the electrical polarization induced by displacement of atoms, are key quantities for understanding how deformations and electric fields are coupled in insulating materials. It has been widely assumed that in metals BECs are not relevant, since electrostatic fields are screened by free carriers, and electrical polarization is not well defined.
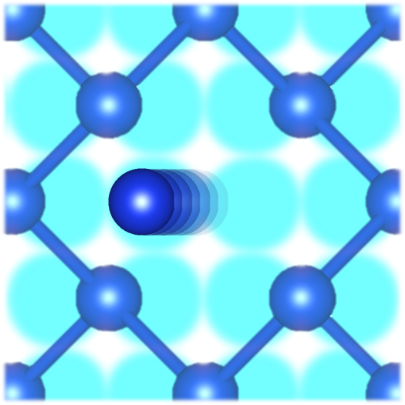
However, complete screening of fields only occurs if we assume that electrons move adiabatically with ionic displacements (i.e., the Born-Oppenheimer approximation). We show in this work that going beyond the adiabatic approximation results in nonadiabatic BECs that are well-defined and finite, even in the low-frequency regime relevant for lattice dynamics.
In addition, we show that the sum of nonadiabatic BECs over the atoms in a unit cell of the crystal no longer vanishes, as it must in insulators. We derive a generalized sum rule, demonstrating that the sum of nonadiabatic BECs equals the Drude weight, i.e., the density of free electrons available for conduction.
This sum rule is demonstrated by first-principles calculations. This work extends the concept of dynamical charges to metals and doped semiconductors as well as providing a novel physical interpretation of the Drude weight in terms of lattice dynamics, instead of transport.